Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 77655 by TawaTawa last updated on 08/Jan/20

Find the remainder when x + x^(25) + x^(49) + x^(81) is divided by x^3 − 1

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{remainder}\:\mathrm{when}\:\:\:\:\mathrm{x}\:+\:\mathrm{x}^{\mathrm{25}} \:+\:\mathrm{x}^{\mathrm{49}} \:+\:\mathrm{x}^{\mathrm{81}} \:\:\mathrm{is}\:\mathrm{divided} \\ $$$$\mathrm{by}\:\:\mathrm{x}^{\mathrm{3}} \:−\:\mathrm{1} \\ $$

Commented by jagoll last updated on 08/Jan/20

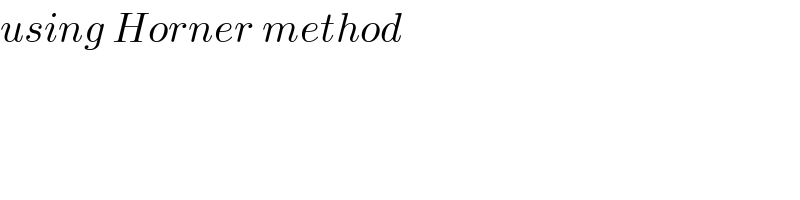
using Horner method

$${using}\:{Horner}\:{method} \\ $$

Answered by mind is power last updated on 09/Jan/20

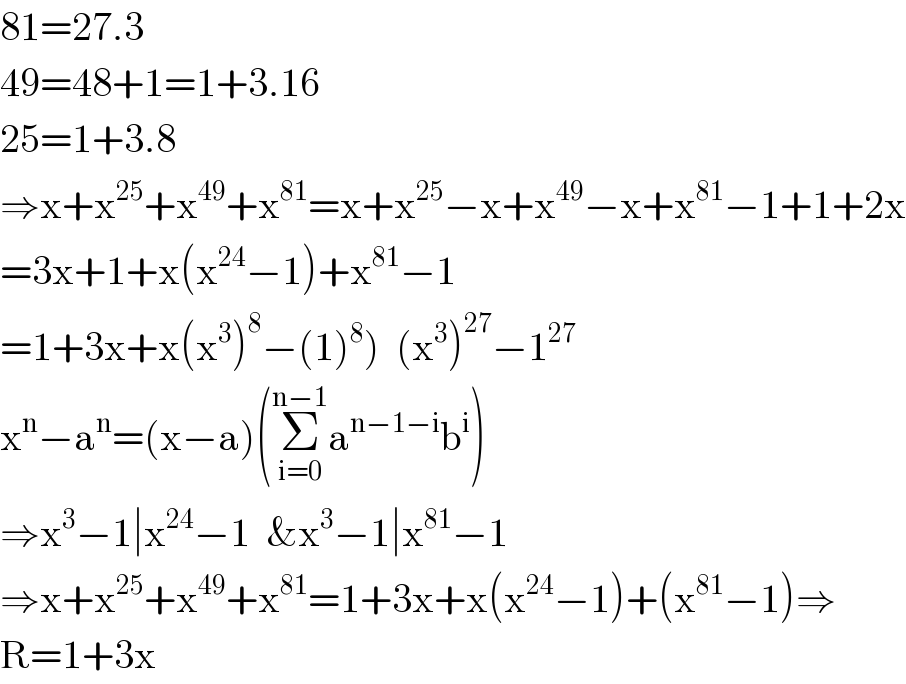
81=27.3 49=48+1=1+3.16 25=1+3.8 ⇒x+x^(25) +x^(49) +x^(81) =x+x^(25) −x+x^(49) −x+x^(81) −1+1+2x =3x+1+x(x^(24) −1)+x^(81) −1 =1+3x+x(x^3 )^8 −(1)^8 ) (x^3 )^(27) −1^(27) x^n −a^n =(x−a)(Σ_(i=0) ^(n−1) a^(n−1−i) b^i ) ⇒x^3 −1∣x^(24) −1 &x^3 −1∣x^(81) −1 ⇒x+x^(25) +x^(49) +x^(81) =1+3x+x(x^(24) −1)+(x^(81) −1)⇒ R=1+3x

$$\mathrm{81}=\mathrm{27}.\mathrm{3} \\ $$$$\mathrm{49}=\mathrm{48}+\mathrm{1}=\mathrm{1}+\mathrm{3}.\mathrm{16} \\ $$$$\mathrm{25}=\mathrm{1}+\mathrm{3}.\mathrm{8} \\ $$$$\Rightarrow\mathrm{x}+\mathrm{x}^{\mathrm{25}} +\mathrm{x}^{\mathrm{49}} +\mathrm{x}^{\mathrm{81}} =\mathrm{x}+\mathrm{x}^{\mathrm{25}} −\mathrm{x}+\mathrm{x}^{\mathrm{49}} −\mathrm{x}+\mathrm{x}^{\mathrm{81}} −\mathrm{1}+\mathrm{1}+\mathrm{2x} \\ $$$$=\mathrm{3x}+\mathrm{1}+\mathrm{x}\left(\mathrm{x}^{\mathrm{24}} −\mathrm{1}\right)+\mathrm{x}^{\mathrm{81}} −\mathrm{1} \\ $$$$\left.=\mathrm{1}+\mathrm{3x}+\mathrm{x}\left(\mathrm{x}^{\mathrm{3}} \right)^{\mathrm{8}} −\left(\mathrm{1}\right)^{\mathrm{8}} \right)\:\:\left(\mathrm{x}^{\mathrm{3}} \right)^{\mathrm{27}} −\mathrm{1}^{\mathrm{27}} \\ $$$$\mathrm{x}^{\mathrm{n}} −\mathrm{a}^{\mathrm{n}} =\left(\mathrm{x}−\mathrm{a}\right)\left(\underset{\mathrm{i}=\mathrm{0}} {\overset{\mathrm{n}−\mathrm{1}} {\sum}}\mathrm{a}^{\mathrm{n}−\mathrm{1}−\mathrm{i}} \mathrm{b}^{\mathrm{i}} \right) \\ $$$$\Rightarrow\mathrm{x}^{\mathrm{3}} −\mathrm{1}\mid\mathrm{x}^{\mathrm{24}} −\mathrm{1}\:\:\&\mathrm{x}^{\mathrm{3}} −\mathrm{1}\mid\mathrm{x}^{\mathrm{81}} −\mathrm{1} \\ $$$$\Rightarrow\mathrm{x}+\mathrm{x}^{\mathrm{25}} +\mathrm{x}^{\mathrm{49}} +\mathrm{x}^{\mathrm{81}} =\mathrm{1}+\mathrm{3x}+\mathrm{x}\left(\mathrm{x}^{\mathrm{24}} −\mathrm{1}\right)+\left(\mathrm{x}^{\mathrm{81}} −\mathrm{1}\right)\Rightarrow \\ $$$$\mathrm{R}=\mathrm{1}+\mathrm{3x} \\ $$

Commented by TawaTawa last updated on 09/Jan/20

God bless you sir

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Answered by john santu last updated on 09/Jan/20

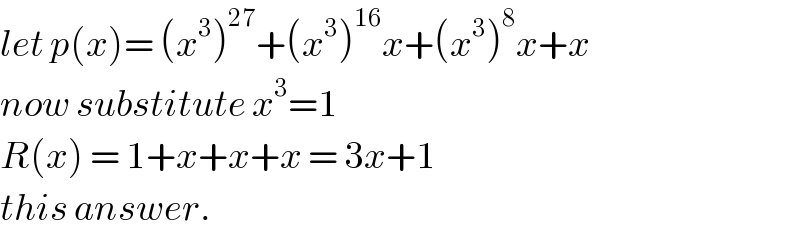
let p(x)= (x^3 )^(27) +(x^3 )^(16) x+(x^3 )^8 x+x now substitute x^3 =1 R(x) = 1+x+x+x = 3x+1 this answer.

$${let}\:{p}\left({x}\right)=\:\left({x}^{\mathrm{3}} \right)^{\mathrm{27}} +\left({x}^{\mathrm{3}} \right)^{\mathrm{16}} {x}+\left({x}^{\mathrm{3}} \right)^{\mathrm{8}} {x}+{x} \\ $$$${now}\:{substitute}\:{x}^{\mathrm{3}} =\mathrm{1} \\ $$$${R}\left({x}\right)\:=\:\mathrm{1}+{x}+{x}+{x}\:=\:\mathrm{3}{x}+\mathrm{1} \\ $$$${this}\:{answer}.\: \\ $$

Commented by mind is power last updated on 09/Jan/20

isee now ididnt see this methode befor lets proves this if P(x)=x^n , Q(x)=x^m +t n≥m let R remainder P over Q first n=m.a+r ∃!(a,r) euclidienns division x^(ma+r) =x^r (x^m )^a ,x^m =Q(x)−t x^r .(x^m )^a =x^r (Q(x)−t)^a by newtoon Methode (Q(x)−t)^a =Σ_(k=0) ^n Q(X)^k (−t)^(a−k) =(−t)^a +Σ_(k=1) ^n (Q(x))^k (−t)^(a−k) ,∀k≥1 Q(x)∣Q(x)^k (−t)^(a−k) ⇒ (Q(x)−t))^a =(−t)^a +Q(x).S(x) ⇒x^r .(x^m )^a =X^r ((−t)^a +QS(x)) =(−t)^a X^r +X^r S(X).Q(X) reminder is (−t)^a X^r How to use it reminder X^(1000) ,by X^7 +12 1000=n,m=7,t=12 1000=7.142+6,a=142,r=6 R(x)=(−12)^(142) .X^6 if P(x)=Σ_(k=0) ^n a_k X^k ,use sam idee for every K and sum the answer

$$\mathrm{isee}\: \\ $$$$\mathrm{now}\:\mathrm{ididnt}\:\mathrm{see}\:\mathrm{this}\:\mathrm{methode}\:\mathrm{befor} \\ $$$$\mathrm{lets}\:\mathrm{proves}\:\mathrm{this} \\ $$$$\mathrm{if}\:\mathrm{P}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{n}} ,\:\:\:\mathrm{Q}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{m}} +\mathrm{t} \\ $$$$\mathrm{n}\geqslant\mathrm{m}\:\:\mathrm{let}\:\mathrm{R}\:\mathrm{remainder}\:\:\mathrm{P}\:\mathrm{over}\:\mathrm{Q} \\ $$$$\mathrm{first}\:\mathrm{n}=\mathrm{m}.\mathrm{a}+\mathrm{r}\:\:\exists!\left(\mathrm{a},\mathrm{r}\right)\:\mathrm{euclidienns}\:\mathrm{division} \\ $$$$\mathrm{x}^{\mathrm{ma}+\mathrm{r}} =\mathrm{x}^{\mathrm{r}} \left(\mathrm{x}^{\mathrm{m}} \right)^{\mathrm{a}} ,\mathrm{x}^{\mathrm{m}} =\mathrm{Q}\left(\mathrm{x}\right)−\mathrm{t} \\ $$$$\mathrm{x}^{\mathrm{r}} .\left(\mathrm{x}^{\mathrm{m}} \right)^{\mathrm{a}} =\mathrm{x}^{\mathrm{r}} \left(\mathrm{Q}\left(\mathrm{x}\right)−\mathrm{t}\right)^{\mathrm{a}} \\ $$$$\mathrm{by}\:\mathrm{newtoon}\:\mathrm{Methode} \\ $$$$\left(\mathrm{Q}\left(\mathrm{x}\right)−\mathrm{t}\right)^{\mathrm{a}} =\underset{\mathrm{k}=\mathrm{0}} {\overset{\mathrm{n}} {\sum}}\mathrm{Q}\left(\mathrm{X}\right)^{\mathrm{k}} \left(−\mathrm{t}\right)^{\mathrm{a}−\mathrm{k}} =\left(−\mathrm{t}\right)^{\mathrm{a}} +\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\left(\mathrm{Q}\left(\mathrm{x}\right)\right)^{\mathrm{k}} \left(−\mathrm{t}\right)^{\mathrm{a}−\mathrm{k}} \\ $$$$,\forall\mathrm{k}\geqslant\mathrm{1}\:\:\mathrm{Q}\left(\mathrm{x}\right)\mid\mathrm{Q}\left(\mathrm{x}\right)^{\mathrm{k}} \left(−\mathrm{t}\right)^{\mathrm{a}−\mathrm{k}} \Rightarrow \\ $$$$\left.\left(\mathrm{Q}\left(\mathrm{x}\right)−\mathrm{t}\right)\right)^{\mathrm{a}} =\left(−\mathrm{t}\right)^{\mathrm{a}} +\mathrm{Q}\left(\mathrm{x}\right).\mathrm{S}\left(\mathrm{x}\right) \\ $$$$\Rightarrow\mathrm{x}^{\mathrm{r}} .\left(\mathrm{x}^{\mathrm{m}} \right)^{\mathrm{a}} =\mathrm{X}^{\mathrm{r}} \left(\left(−\mathrm{t}\right)^{\mathrm{a}} +\mathrm{QS}\left(\mathrm{x}\right)\right) \\ $$$$=\left(−\mathrm{t}\right)^{\mathrm{a}} \mathrm{X}^{\mathrm{r}} +\mathrm{X}^{\mathrm{r}} \mathrm{S}\left(\mathrm{X}\right).\mathrm{Q}\left(\mathrm{X}\right)\:\:\mathrm{reminder}\:\mathrm{is}\:\:\:\left(−\mathrm{t}\right)^{\mathrm{a}} \mathrm{X}^{\mathrm{r}} \\ $$$$\mathrm{How}\:\mathrm{to}\:\mathrm{use}\:\mathrm{it}\: \\ $$$$\mathrm{reminder}\:\mathrm{X}^{\mathrm{1000}} ,\mathrm{by}\:\mathrm{X}^{\mathrm{7}} +\mathrm{12} \\ $$$$\mathrm{1000}=\mathrm{n},\mathrm{m}=\mathrm{7},\mathrm{t}=\mathrm{12} \\ $$$$\mathrm{1000}=\mathrm{7}.\mathrm{142}+\mathrm{6},\mathrm{a}=\mathrm{142},\mathrm{r}=\mathrm{6} \\ $$$$\mathrm{R}\left(\mathrm{x}\right)=\left(−\mathrm{12}\right)^{\mathrm{142}} .\mathrm{X}^{\mathrm{6}} \\ $$$$\mathrm{if}\:\mathrm{P}\left(\mathrm{x}\right)=\underset{\mathrm{k}=\mathrm{0}} {\overset{\mathrm{n}} {\sum}}\mathrm{a}_{\mathrm{k}} \mathrm{X}^{\mathrm{k}} ,\mathrm{use}\:\mathrm{sam}\:\mathrm{idee}\:\mathrm{for}\:\mathrm{every}\:\mathrm{K}\:\mathrm{and}\:\mathrm{sum}\:\mathrm{the}\:\mathrm{answer} \\ $$$$ \\ $$

Commented by john santu last updated on 09/Jan/20

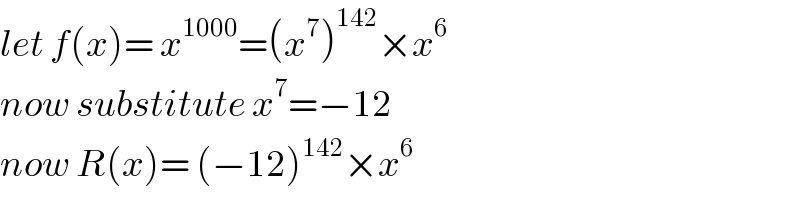
let f(x)= x^(1000) =(x^7 )^(142) ×x^6 now substitute x^7 =−12 now R(x)= (−12)^(142) ×x^6

$${let}\:{f}\left({x}\right)=\:{x}^{\mathrm{1000}} =\left({x}^{\mathrm{7}} \right)^{\mathrm{142}} ×{x}^{\mathrm{6}} \\ $$$${now}\:{substitute}\:{x}^{\mathrm{7}} =−\mathrm{12} \\ $$$${now}\:{R}\left({x}\right)=\:\left(−\mathrm{12}\right)^{\mathrm{142}} ×{x}^{\mathrm{6}} \\ $$

Commented by TawaTawa last updated on 09/Jan/20

God bless you sir

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com