Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 80983 by jagoll last updated on 08/Feb/20

if lim_(x→0) ((ae^x −bsin x+ce^(−x) )/(xsin x)) = 2 what is a+b+c ?

$${if}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{ae}^{{x}} −{b}\mathrm{sin}\:{x}+{ce}^{−{x}} }{{x}\mathrm{sin}\:{x}}\:=\:\mathrm{2} \\ $$$${what}\:{is}\:{a}+{b}+{c}\:? \\ $$

Commented by jagoll last updated on 09/Feb/20

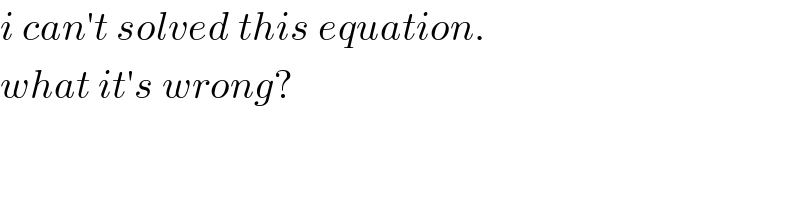
i can′t solved this equation. what it′s wrong?

$${i}\:{can}'{t}\:{solved}\:{this}\:{equation}.\: \\ $$$${what}\:{it}'{s}\:{wrong}? \\ $$

Commented by jagoll last updated on 09/Feb/20

step (1) limit must be (0/0) ⇒a +c = 0 ⇒c = −a step(2) lim_(x→0) ((ae^x −bsin x −ae^(−x) )/(xsin x)) =2 L ^� hopital rule lim_(x→0) ((ae^x −bcos x+ae^(−x) )/(sin x+xcos x)) = 2 since denumerator = 0 , ⇒2a −b = 0 ⇒b = 2a L ^� hopital again lim_(x→0) ((ae^x +bsin x−ae^(−x) )/(2cos x−xsin x))=2 ⇒ (0/2)=2 ⇒?

$${step}\:\left(\mathrm{1}\right)\:{limit}\:{must}\:{be}\:\frac{\mathrm{0}}{\mathrm{0}} \\ $$$$\Rightarrow{a}\:+{c}\:=\:\mathrm{0}\:\Rightarrow{c}\:=\:−{a} \\ $$$${step}\left(\mathrm{2}\right)\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{ae}^{{x}} −{b}\mathrm{sin}\:{x}\:−{ae}^{−{x}} }{{x}\mathrm{sin}\:{x}}\:=\mathrm{2} \\ $$$${L}\hat {\:}{hopital}\:{rule} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{ae}^{{x}} −{b}\mathrm{cos}\:{x}+{ae}^{−{x}} }{\mathrm{sin}\:{x}+{x}\mathrm{cos}\:{x}}\:=\:\mathrm{2} \\ $$$${since}\:{denumerator}\:=\:\mathrm{0}\:,\: \\ $$$$\Rightarrow\mathrm{2}{a}\:−{b}\:=\:\mathrm{0}\:\Rightarrow{b}\:=\:\mathrm{2}{a} \\ $$$${L}\hat {\:}{hopital}\:{again} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{ae}^{{x}} +{b}\mathrm{sin}\:{x}−{ae}^{−{x}} }{\mathrm{2cos}\:{x}−{x}\mathrm{sin}\:{x}}=\mathrm{2} \\ $$$$\Rightarrow\:\frac{\mathrm{0}}{\mathrm{2}}=\mathrm{2}\:\Rightarrow? \\ $$

Commented by abdomathmax last updated on 09/Feb/20

⇒lim_(x→0) ((ae^x −bsinx +c e^(−x) )/(xsinx))−2=0 ⇒ lim_(x→0) ((a e^x −bsinx+ce^(−x) −2xsinx)/(xsinx))=0⇒ lim_(x→0) u(x)==lim_(x→0) u^′ (x)=0 and lim_(x→0) v(x)=lim_(x→0) v^′ (x)=0 with u(x)=ae^x −bsinx +ce^(−x) −2xsinx ⇒ u(0)=0 ⇒a +c =0 ⇒c=−a u^′ (x)=ae^x −bcosx−ce^(−x) −2sinx−2x cosx u^′ (0)=0 ⇒a−b−c=0 ⇒2a−b=0 ⇒b=2a ⇒a+b+c =2(b+c)=2(2a−a)=2a if we get a=1 ⇒a+b+c=2

$$\Rightarrow{lim}_{{x}\rightarrow\mathrm{0}} \:\:\frac{{ae}^{{x}} −{bsinx}\:+{c}\:{e}^{−{x}} }{{xsinx}}−\mathrm{2}=\mathrm{0}\:\Rightarrow \\ $$$${lim}_{{x}\rightarrow\mathrm{0}} \:\:\:\frac{{a}\:{e}^{{x}} −{bsinx}+{ce}^{−{x}} −\mathrm{2}{xsinx}}{{xsinx}}=\mathrm{0}\Rightarrow \\ $$$${lim}_{{x}\rightarrow\mathrm{0}} {u}\left({x}\right)=={lim}_{{x}\rightarrow\mathrm{0}} {u}^{'} \left({x}\right)=\mathrm{0}\:{and} \\ $$$${lim}_{{x}\rightarrow\mathrm{0}} {v}\left({x}\right)={lim}_{{x}\rightarrow\mathrm{0}} \:\:{v}^{'} \left({x}\right)=\mathrm{0}\:{with} \\ $$$${u}\left({x}\right)={ae}^{{x}} −{bsinx}\:+{ce}^{−{x}} −\mathrm{2}{xsinx}\:\Rightarrow \\ $$$${u}\left(\mathrm{0}\right)=\mathrm{0}\:\Rightarrow{a}\:+{c}\:=\mathrm{0}\:\Rightarrow{c}=−{a} \\ $$$${u}^{'} \left({x}\right)={ae}^{{x}} −{bcosx}−{ce}^{−{x}} −\mathrm{2}{sinx}−\mathrm{2}{x}\:{cosx} \\ $$$${u}^{'} \left(\mathrm{0}\right)=\mathrm{0}\:\Rightarrow{a}−{b}−{c}=\mathrm{0}\:\Rightarrow\mathrm{2}{a}−{b}=\mathrm{0}\:\Rightarrow{b}=\mathrm{2}{a} \\ $$$$\Rightarrow{a}+{b}+{c}\:=\mathrm{2}\left({b}+{c}\right)=\mathrm{2}\left(\mathrm{2}{a}−{a}\right)=\mathrm{2}{a} \\ $$$${if}\:{we}\:{get}\:{a}=\mathrm{1}\:\Rightarrow{a}+{b}+{c}=\mathrm{2} \\ $$

Commented by john santu last updated on 09/Feb/20

you are right sir

$${you}\:{are}\:{right}\:{sir} \\ $$

Commented by jagoll last updated on 09/Feb/20

how to get a = 1 ?

$${how}\:{to}\:{get}\:{a}\:=\:\mathrm{1}\:? \\ $$

Commented by jagoll last updated on 09/Feb/20

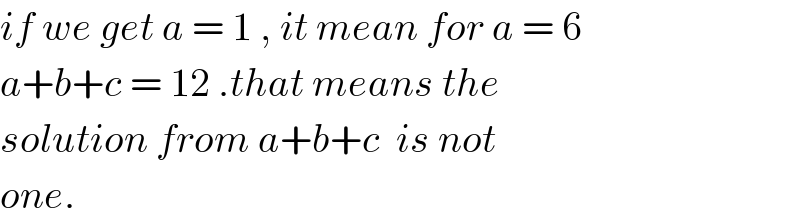
if we get a = 1 , it mean for a = 6 a+b+c = 12 .that means the solution from a+b+c is not one.

$${if}\:{we}\:{get}\:{a}\:=\:\mathrm{1}\:,\:{it}\:{mean}\:{for}\:{a}\:=\:\mathrm{6} \\ $$$${a}+{b}+{c}\:=\:\mathrm{12}\:.{that}\:{means}\:{the} \\ $$$${solution}\:{from}\:{a}+{b}+{c}\:\:{is}\:{not}\: \\ $$$${one}. \\ $$

Commented by mr W last updated on 09/Feb/20

have you checked sir? i don′t think the answer is correct. example: a=1,b=2,c=−1. lim_(x→0) ((e^x −2sin x−e^(−x) )/(xsin x)) =lim_(x→0) ((e^x −2cos x+e^(−x) )/(sin x+x cos x)) =lim_(x→0) ((e^x +2sin x−e^(−x) )/(2 cos x−x sin x)) =(0/2)=0≠2 my answer is: there exist no values for a,b, c such that lim_(x→0) ((ae^x −bsin x+ce^(−x) )/(xsin x))=2.

$${have}\:{you}\:{checked}\:{sir}? \\ $$$${i}\:{don}'{t}\:{think}\:{the}\:{answer}\:{is}\:{correct}. \\ $$$${example}:\:{a}=\mathrm{1},{b}=\mathrm{2},{c}=−\mathrm{1}. \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{x}} −\mathrm{2sin}\:{x}−{e}^{−{x}} }{{x}\mathrm{sin}\:{x}} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{x}} −\mathrm{2cos}\:{x}+{e}^{−{x}} }{\mathrm{sin}\:{x}+{x}\:\mathrm{cos}\:{x}} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{e}^{{x}} +\mathrm{2sin}\:{x}−{e}^{−{x}} }{\mathrm{2}\:\mathrm{cos}\:{x}−{x}\:\mathrm{sin}\:{x}} \\ $$$$=\frac{\mathrm{0}}{\mathrm{2}}=\mathrm{0}\neq\mathrm{2} \\ $$$$ \\ $$$${my}\:{answer}\:{is}: \\ $$$${there}\:{exist}\:{no}\:{values}\:{for}\:{a},{b},\:{c}\:{such} \\ $$$${that}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{ae}^{{x}} −{b}\mathrm{sin}\:{x}+{ce}^{−{x}} }{{x}\mathrm{sin}\:{x}}=\mathrm{2}. \\ $$

Commented by jagoll last updated on 09/Feb/20

yes sir. i think it question not right

$${yes}\:{sir}.\:{i}\:{think}\:{it}\:{question}\:{not}\:{right} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com