
Question Number 85801 by mathmax by abdo last updated on 24/Mar/20
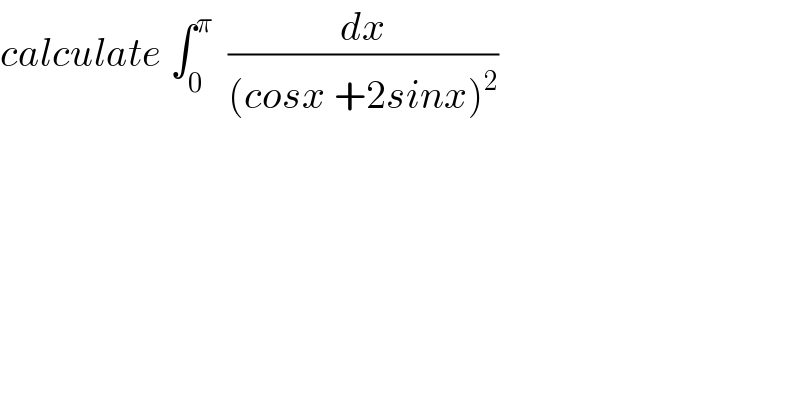
$${calculate}\:\int_{\mathrm{0}} ^{\pi} \:\:\frac{{dx}}{\left({cosx}\:+\mathrm{2}{sinx}\right)^{\mathrm{2}} } \\ $$
Commented by john santu last updated on 25/Mar/20
![(cos x+2sin x)^2 = 5 cos^2 (x−tan^(−1) (2)) ∫ (dx/(5 cos^2 (x−tan^(−1) (2)))) = (1/5) tan (x−tan^(−1) (2)) = (1/5)(((tan x−2)/(1+2tan x))) +c so ⇒ ∫_0 ^π (dx/((cos x+2sin x)^2 )) = (1/5) [ ((tan x−2)/(1+2tan x)) ]_( 0) ^( π) = (1/5) [ −2 −(−2) ] = 0](Q85803.png)
$$\left(\mathrm{cos}\:{x}+\mathrm{2sin}\:{x}\right)^{\mathrm{2}} \:=\:\mathrm{5}\:\mathrm{cos}\:^{\mathrm{2}} \left({x}−\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}\right)\right) \\ $$$$\int\:\frac{{dx}}{\mathrm{5}\:\mathrm{cos}\:^{\mathrm{2}} \left({x}−\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}\right)\right)}\:= \\ $$$$\frac{\mathrm{1}}{\mathrm{5}}\:\mathrm{tan}\:\left({x}−\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}\right)\right)\:=\: \\ $$$$\frac{\mathrm{1}}{\mathrm{5}}\left(\frac{\mathrm{tan}\:{x}−\mathrm{2}}{\mathrm{1}+\mathrm{2tan}\:{x}}\right)\:+{c} \\ $$$${so}\:\Rightarrow\:\underset{\mathrm{0}} {\overset{\pi} {\int}}\:\frac{{dx}}{\left(\mathrm{cos}\:{x}+\mathrm{2sin}\:{x}\right)^{\mathrm{2}} }\:=\: \\ $$$$\frac{\mathrm{1}}{\mathrm{5}}\:\left[\:\frac{\mathrm{tan}\:{x}−\mathrm{2}}{\mathrm{1}+\mathrm{2tan}\:{x}}\:\underset{\:\mathrm{0}} {\overset{\:\:\pi} {\right]}}\:=\: \\ $$$$\frac{\mathrm{1}}{\mathrm{5}}\:\left[\:−\mathrm{2}\:−\left(−\mathrm{2}\right)\:\right]\:=\:\mathrm{0} \\ $$
Commented by mathmax by abdo last updated on 26/Mar/20
![et A =∫_0 ^π (dx/((cosx+2sinx)^2 )) changement tan((x/2))=t give A =∫_0 ^∞ ((2dt)/((1+t^2 ){((1−t^2 )/(1+t^2 ))+((4t)/(1+t^2 ))}^2 )) =∫_0 ^∞ ((2(1+t^2 ))/((−t^2 +4t+1)^2 ))dt =2∫_0 ^∞ ((t^2 +1)/((t^2 −4t−1)^2 ))dt let decompose F(t)=((t^2 +1)/((t^2 −4t−1)^2 )) t^2 −4t−1 =0→Δ^′ =4+1 =5 ⇒t_1 =2+(√5) and t_2 =2−(√5) F(t) =((t^2 +1)/((t−t_1 )^2 (t−t_2 )^2 )) =(a/(t−t_1 )) +(b/((t−t_1 )^2 )) +(c/(t−t_2 )) +(d/((t−t_2 )^2 )) b =((t_1 ^2 +1)/((t_1 −t_2 )^2 )) =((4+4(√5)+5+1)/((2(√5))^2 )) =((10+4(√5))/(20)) =((5+2(√5))/(10)) d =((t_2 ^2 +1)/((t_2 −t_1 )^2 )) =((4−4(√5)+5+1)/(20)) =((10−4(√5))/(20)) =((5−2(√5))/(10)) lim_(t→+∞) tF(t) =0 =a+c ⇒c=−a F(0)=(1/((t_1 t_2 )^2 )) =1 =−(a/t_1 ) +(b/t_1 ^2 ) +(a/t_2 ) +(d/t_2 ^2 ) ⇒ ((1/t_2 )−(1/t_1 ))a =1−(b/t_1 ^2 )−(d/t_2 ^2 ) ⇒(((2(√5))/(−1)))a =1−(b/(4+4(√5)+5))−(d/(4−4(√5)+5)) =1−(b/(9+4(√5)))−(d/(9−4(√5))) ⇒a =−(1/(2(√5))){1−(b/(9+4(√5)))−(d/(9−4(√5)))} ⇒ A =2a∫_0 ^∞ (dt/(t−t_1 )) +2b∫_0 ^∞ (dt/((t−t_1 )^2 )) +2c ∫_0 ^∞ (dt/(t−t_2 )) +2d∫_0 ^∞ (dt/((t−t_2 )^2 )) =[2aln∣t−t_1 ∣+2cln∣t−t_2 ∣]_0 ^(+∞) +[((−2b)/(t−t_1 ))−((2d)/(t−t_2 ))]_0 ^(+∞) rest to finish the calculus....](Q85968.png)
$${et}\:{A}\:=\int_{\mathrm{0}} ^{\pi} \:\:\frac{{dx}}{\left({cosx}+\mathrm{2}{sinx}\right)^{\mathrm{2}} }\:\:{changement}\:{tan}\left(\frac{{x}}{\mathrm{2}}\right)={t}\:{give} \\ $$$${A}\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{\mathrm{2}{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\left\{\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }+\frac{\mathrm{4}{t}}{\mathrm{1}+{t}^{\mathrm{2}} }\right\}^{\mathrm{2}} }\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{\left(−{t}^{\mathrm{2}} +\mathrm{4}{t}+\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{\mathrm{2}} +\mathrm{1}}{\left({t}^{\mathrm{2}} −\mathrm{4}{t}−\mathrm{1}\right)^{\mathrm{2}} }{dt}\:\:{let}\:{decompose}\:{F}\left({t}\right)=\frac{{t}^{\mathrm{2}} \:+\mathrm{1}}{\left({t}^{\mathrm{2}} −\mathrm{4}{t}−\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${t}^{\mathrm{2}} −\mathrm{4}{t}−\mathrm{1}\:=\mathrm{0}\rightarrow\Delta^{'} =\mathrm{4}+\mathrm{1}\:=\mathrm{5}\:\Rightarrow{t}_{\mathrm{1}} =\mathrm{2}+\sqrt{\mathrm{5}}\:{and}\:{t}_{\mathrm{2}} =\mathrm{2}−\sqrt{\mathrm{5}} \\ $$$${F}\left({t}\right)\:=\frac{{t}^{\mathrm{2}} \:+\mathrm{1}}{\left({t}−{t}_{\mathrm{1}} \right)^{\mathrm{2}} \left({t}−{t}_{\mathrm{2}} \right)^{\mathrm{2}} }\:=\frac{{a}}{{t}−{t}_{\mathrm{1}} }\:+\frac{{b}}{\left({t}−{t}_{\mathrm{1}} \right)^{\mathrm{2}} }\:+\frac{{c}}{{t}−{t}_{\mathrm{2}} }\:+\frac{{d}}{\left({t}−{t}_{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$$${b}\:=\frac{{t}_{\mathrm{1}} ^{\mathrm{2}} +\mathrm{1}}{\left({t}_{\mathrm{1}} −{t}_{\mathrm{2}} \right)^{\mathrm{2}} }\:=\frac{\mathrm{4}+\mathrm{4}\sqrt{\mathrm{5}}+\mathrm{5}+\mathrm{1}}{\left(\mathrm{2}\sqrt{\mathrm{5}}\right)^{\mathrm{2}} }\:=\frac{\mathrm{10}+\mathrm{4}\sqrt{\mathrm{5}}}{\mathrm{20}}\:=\frac{\mathrm{5}+\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{10}} \\ $$$${d}\:=\frac{{t}_{\mathrm{2}} ^{\mathrm{2}} \:+\mathrm{1}}{\left({t}_{\mathrm{2}} −{t}_{\mathrm{1}} \right)^{\mathrm{2}} }\:=\frac{\mathrm{4}−\mathrm{4}\sqrt{\mathrm{5}}+\mathrm{5}+\mathrm{1}}{\mathrm{20}}\:=\frac{\mathrm{10}−\mathrm{4}\sqrt{\mathrm{5}}}{\mathrm{20}}\:=\frac{\mathrm{5}−\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{10}} \\ $$$${lim}_{{t}\rightarrow+\infty} {tF}\left({t}\right)\:=\mathrm{0}\:={a}+{c}\:\Rightarrow{c}=−{a} \\ $$$${F}\left(\mathrm{0}\right)=\frac{\mathrm{1}}{\left({t}_{\mathrm{1}} {t}_{\mathrm{2}} \right)^{\mathrm{2}} }\:=\mathrm{1}\:=−\frac{{a}}{{t}_{\mathrm{1}} }\:+\frac{{b}}{{t}_{\mathrm{1}} ^{\mathrm{2}} }\:+\frac{{a}}{{t}_{\mathrm{2}} }\:+\frac{{d}}{{t}_{\mathrm{2}} ^{\mathrm{2}} }\:\Rightarrow \\ $$$$\left(\frac{\mathrm{1}}{{t}_{\mathrm{2}} }−\frac{\mathrm{1}}{{t}_{\mathrm{1}} }\right){a}\:=\mathrm{1}−\frac{{b}}{{t}_{\mathrm{1}} ^{\mathrm{2}} }−\frac{{d}}{{t}_{\mathrm{2}} ^{\mathrm{2}} }\:\Rightarrow\left(\frac{\mathrm{2}\sqrt{\mathrm{5}}}{−\mathrm{1}}\right){a}\:=\mathrm{1}−\frac{{b}}{\mathrm{4}+\mathrm{4}\sqrt{\mathrm{5}}+\mathrm{5}}−\frac{{d}}{\mathrm{4}−\mathrm{4}\sqrt{\mathrm{5}}+\mathrm{5}} \\ $$$$=\mathrm{1}−\frac{{b}}{\mathrm{9}+\mathrm{4}\sqrt{\mathrm{5}}}−\frac{{d}}{\mathrm{9}−\mathrm{4}\sqrt{\mathrm{5}}}\:\Rightarrow{a}\:=−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{5}}}\left\{\mathrm{1}−\frac{{b}}{\mathrm{9}+\mathrm{4}\sqrt{\mathrm{5}}}−\frac{{d}}{\mathrm{9}−\mathrm{4}\sqrt{\mathrm{5}}}\right\}\:\Rightarrow \\ $$$${A}\:=\mathrm{2}{a}\int_{\mathrm{0}} ^{\infty} \frac{{dt}}{{t}−{t}_{\mathrm{1}} }\:+\mathrm{2}{b}\int_{\mathrm{0}} ^{\infty} \:\frac{{dt}}{\left({t}−{t}_{\mathrm{1}} \right)^{\mathrm{2}} }\:+\mathrm{2}{c}\:\int_{\mathrm{0}} ^{\infty} \frac{{dt}}{{t}−{t}_{\mathrm{2}} }\:+\mathrm{2}{d}\int_{\mathrm{0}} ^{\infty} \:\frac{{dt}}{\left({t}−{t}_{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$$$=\left[\mathrm{2}{aln}\mid{t}−{t}_{\mathrm{1}} \mid+\mathrm{2}{cln}\mid{t}−{t}_{\mathrm{2}} \mid\right]_{\mathrm{0}} ^{+\infty} \:+\left[\frac{−\mathrm{2}{b}}{{t}−{t}_{\mathrm{1}} }−\frac{\mathrm{2}{d}}{{t}−{t}_{\mathrm{2}} }\right]_{\mathrm{0}} ^{+\infty} \\ $$$${rest}\:{to}\:{finish}\:{the}\:{calculus}.... \\ $$
Commented by mathmax by abdo last updated on 26/Mar/20
![let try another way we have A =2 ∫_0 ^∞ ((t^2 +1)/((t−t_1 )^2 (t−t_2 )^2 )) with t_1 =2+(√5) and t_2 =2−(√5) A =2∫_0 ^∞ ((t^2 +1)/((((t−t_1 )/(t−t_2 )))^2 (t−t_2 )^4 ))dt we do the changement ((t−t_1 )/(t−t_2 )) =u⇒ t−t_1 =ut−ut_2 ⇒(1−u)t =t_1 −ut_2 ⇒t =((t_1 −ut_2 )/(1−u)) ⇒ dt =((−t_2 (1−u)+(t_1 −ut_2 ))/((1−u)^2 )) =((−t_2 +t_2 u+t_1 −ut_2 )/((1−u)^2 )) =((2(√5))/((1−u)^2 )) t−t_2 =((t_1 −ut_2 )/(1−u))−t_2 =((t_1 −ut_2 −t_2 +ut_2 )/(1−u)) =((2(√5))/(1−u)) ⇒ A =2 ∫_(t_1 /t_2 ) ^1 (((((t_1 −ut_2 )/(1−u)))^2 +1)/(u^2 ×(((2(√5))^4 )/((1−u)^4 ))))×((2(√5))/((1−u)^2 ))du A =(2/((2(√5))^3 )) ∫_(t_1 /t_2 ) ^1 (((t_1 −ut_2 )^2 +(1−u)^2 )/u^2 ) du A =(1/(20(√5))) ∫_(t_1 /t_2 ) ^1 ((t_2 ^2 u^2 +2u +t_1 ^2 +u^2 −2u +1)/u^2 )du (t_1 t_2 =−1) 20(√5) A =∫_(t_1 /t_2 ) ^1 (((1+t_2 ^2 )u^2 +t_1 ^2 )/u^2 )du =(1+t_2 ^2 )(1−(t_1 /t_2 )) −t_1 ^2 [(1/u)]_(t_1 /t_2 ) ^1 =(1+t_2 ^2 )((t_2 −t_1 )/t_2 ) −t_1 ^2 (1−(t_2 /t_1 )) ⇒ A=(1/(20(√5))){(1+(2−(√5))^2 )(((−2(√5))/(2−(√5))))−(2+(√5))^2 (((2(√5))/(2+(√5))))}](Q85976.png)
$${let}\:{try}\:{another}\:{way}\:{we}\:{have} \\ $$$${A}\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\infty} \:\frac{{t}^{\mathrm{2}} \:+\mathrm{1}}{\left({t}−{t}_{\mathrm{1}} \right)^{\mathrm{2}} \left({t}−{t}_{\mathrm{2}} \right)^{\mathrm{2}} }\:\:{with}\:{t}_{\mathrm{1}} =\mathrm{2}+\sqrt{\mathrm{5}}\:{and}\:{t}_{\mathrm{2}} =\mathrm{2}−\sqrt{\mathrm{5}} \\ $$$${A}\:=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{\mathrm{2}} \:+\mathrm{1}}{\left(\frac{{t}−{t}_{\mathrm{1}} }{{t}−{t}_{\mathrm{2}} }\right)^{\mathrm{2}} \left({t}−{t}_{\mathrm{2}} \right)^{\mathrm{4}} }{dt}\:\:{we}\:{do}\:{the}\:{changement}\:\frac{{t}−{t}_{\mathrm{1}} }{{t}−{t}_{\mathrm{2}} }\:={u}\Rightarrow \\ $$$${t}−{t}_{\mathrm{1}} ={ut}−{ut}_{\mathrm{2}} \:\Rightarrow\left(\mathrm{1}−{u}\right){t}\:={t}_{\mathrm{1}} −{ut}_{\mathrm{2}} \:\Rightarrow{t}\:=\frac{{t}_{\mathrm{1}} −{ut}_{\mathrm{2}} }{\mathrm{1}−{u}}\:\Rightarrow \\ $$$${dt}\:=\frac{−{t}_{\mathrm{2}} \left(\mathrm{1}−{u}\right)+\left({t}_{\mathrm{1}} −{ut}_{\mathrm{2}} \right)}{\left(\mathrm{1}−{u}\right)^{\mathrm{2}} }\:=\frac{−{t}_{\mathrm{2}} +{t}_{\mathrm{2}} {u}+{t}_{\mathrm{1}} −{ut}_{\mathrm{2}} }{\left(\mathrm{1}−{u}\right)^{\mathrm{2}} }\:=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\left(\mathrm{1}−{u}\right)^{\mathrm{2}} } \\ $$$${t}−{t}_{\mathrm{2}} =\frac{{t}_{\mathrm{1}} −{ut}_{\mathrm{2}} }{\mathrm{1}−{u}}−{t}_{\mathrm{2}} =\frac{{t}_{\mathrm{1}} −{ut}_{\mathrm{2}} −{t}_{\mathrm{2}} +{ut}_{\mathrm{2}} }{\mathrm{1}−{u}}\:=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{1}−{u}}\:\Rightarrow \\ $$$${A}\:=\mathrm{2}\:\int_{\frac{{t}_{\mathrm{1}} }{{t}_{\mathrm{2}} }} ^{\mathrm{1}} \:\:\:\:\frac{\left(\frac{{t}_{\mathrm{1}} −{ut}_{\mathrm{2}} }{\mathrm{1}−{u}}\right)^{\mathrm{2}} \:+\mathrm{1}}{{u}^{\mathrm{2}} ×\frac{\left(\mathrm{2}\sqrt{\mathrm{5}}\right)^{\mathrm{4}} }{\left(\mathrm{1}−{u}\right)^{\mathrm{4}} }}×\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\left(\mathrm{1}−{u}\right)^{\mathrm{2}} }{du} \\ $$$${A}\:=\frac{\mathrm{2}}{\left(\mathrm{2}\sqrt{\mathrm{5}}\right)^{\mathrm{3}} }\:\int_{\frac{{t}_{\mathrm{1}} }{{t}_{\mathrm{2}} }} ^{\mathrm{1}} \:\:\frac{\left({t}_{\mathrm{1}} −{ut}_{\mathrm{2}} \right)^{\mathrm{2}} \:+\left(\mathrm{1}−{u}\right)^{\mathrm{2}} }{{u}^{\mathrm{2}} }\:{du} \\ $$$${A}\:=\frac{\mathrm{1}}{\mathrm{20}\sqrt{\mathrm{5}}}\:\int_{\frac{{t}_{\mathrm{1}} }{{t}_{\mathrm{2}} }} ^{\mathrm{1}} \:\:\frac{{t}_{\mathrm{2}} ^{\mathrm{2}} {u}^{\mathrm{2}} +\mathrm{2}{u}\:+{t}_{\mathrm{1}} ^{\mathrm{2}} \:\:+{u}^{\mathrm{2}} −\mathrm{2}{u}\:+\mathrm{1}}{{u}^{\mathrm{2}} }{du}\:\:\:\left({t}_{\mathrm{1}} {t}_{\mathrm{2}} =−\mathrm{1}\right) \\ $$$$\mathrm{20}\sqrt{\mathrm{5}}\:{A}\:=\int_{\frac{{t}_{\mathrm{1}} }{{t}_{\mathrm{2}} }} ^{\mathrm{1}} \:\:\frac{\left(\mathrm{1}+{t}_{\mathrm{2}} ^{\mathrm{2}} \right){u}^{\mathrm{2}} \:+{t}_{\mathrm{1}} ^{\mathrm{2}} }{{u}^{\mathrm{2}} }{du} \\ $$$$=\left(\mathrm{1}+{t}_{\mathrm{2}} ^{\mathrm{2}} \right)\left(\mathrm{1}−\frac{{t}_{\mathrm{1}} }{{t}_{\mathrm{2}} }\right)\:−{t}_{\mathrm{1}} ^{\mathrm{2}} \left[\frac{\mathrm{1}}{{u}}\right]_{\frac{{t}_{\mathrm{1}} }{{t}_{\mathrm{2}} }} ^{\mathrm{1}} \:\:=\left(\mathrm{1}+{t}_{\mathrm{2}} ^{\mathrm{2}} \right)\frac{{t}_{\mathrm{2}} −{t}_{\mathrm{1}} }{{t}_{\mathrm{2}} }\:−{t}_{\mathrm{1}} ^{\mathrm{2}} \left(\mathrm{1}−\frac{{t}_{\mathrm{2}} }{{t}_{\mathrm{1}} }\right)\:\Rightarrow \\ $$$${A}=\frac{\mathrm{1}}{\mathrm{20}\sqrt{\mathrm{5}}}\left\{\left(\mathrm{1}+\left(\mathrm{2}−\sqrt{\mathrm{5}}\right)^{\mathrm{2}} \right)\left(\frac{−\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{2}−\sqrt{\mathrm{5}}}\right)−\left(\mathrm{2}+\sqrt{\mathrm{5}}\right)^{\mathrm{2}} \left(\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{2}+\sqrt{\mathrm{5}}}\right)\right\} \\ $$$$ \\ $$$$ \\ $$
