Question and Answers Forum

All Questions      Topic List

Arithmetic Questions

Previous in All Question      Next in All Question      

Previous in Arithmetic      Next in Arithmetic      

Question Number 89913 by M±th+et£s last updated on 20/Apr/20

Commented by M±th+et£s last updated on 20/Apr/20

prove that

$${prove}\:{that} \\ $$

Answered by maths mind last updated on 20/Apr/20

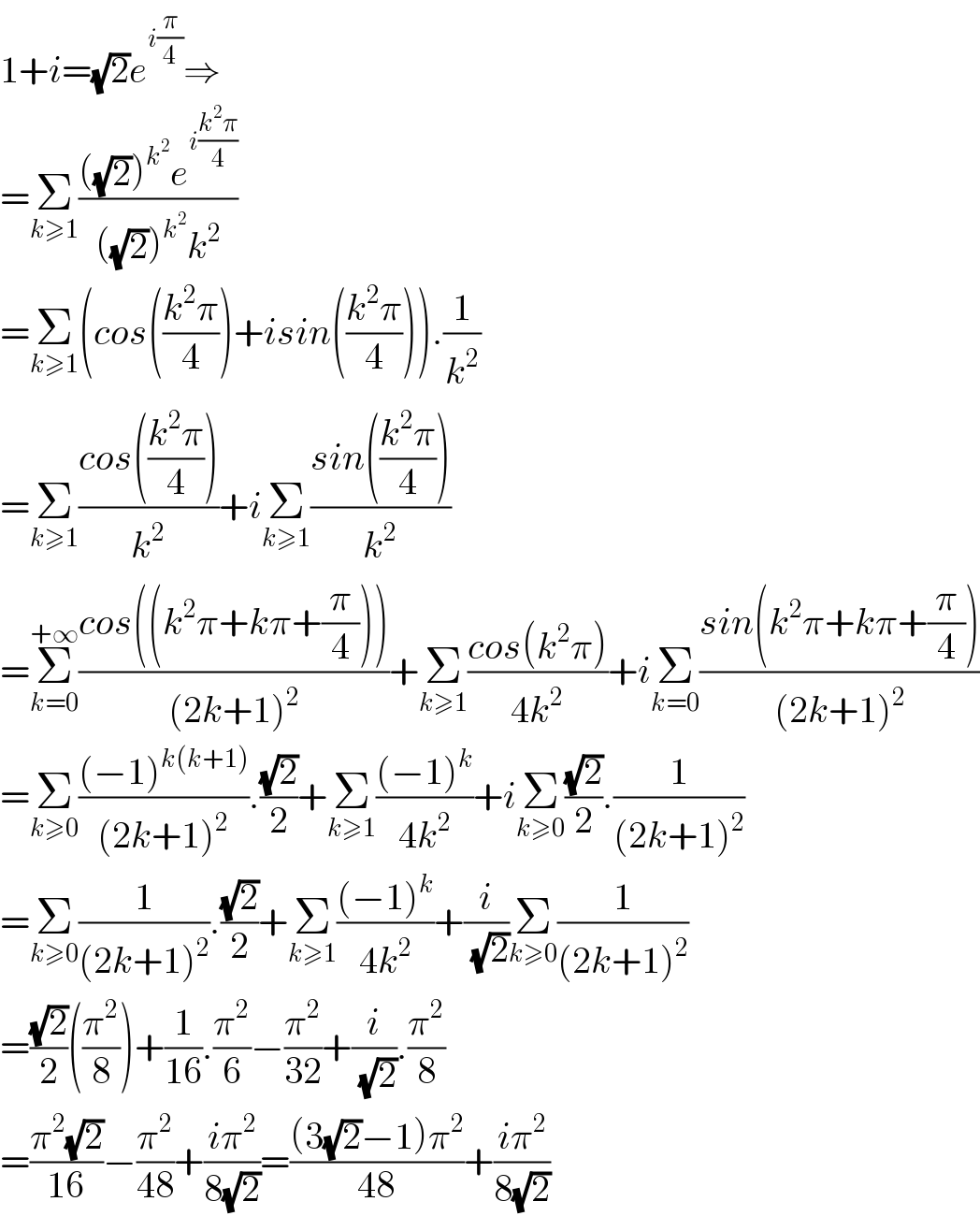
1+i=(√2)e^(i(π/4)) ⇒ =Σ_(k≥1) ((((√2))^k^2 e^(i((k^2 π)/4)) )/(((√2))^k^2 k^2 )) =Σ_(k≥1) (cos(((k^2 π)/4))+isin(((k^2 π)/4))).(1/k^2 ) =Σ_(k≥1) ((cos(((k^2 π)/4)))/k^2 )+iΣ_(k≥1) ((sin(((k^2 π)/4)))/k^2 ) =Σ_(k=0) ^(+∞) ((cos((k^2 π+kπ+(π/4))))/((2k+1)^2 ))+Σ_(k≥1) ((cos(k^2 π))/(4k^2 ))+iΣ_(k=0) ((sin(k^2 π+kπ+(π/4)))/((2k+1)^2 )) =Σ_(k≥0) (((−1)^(k(k+1)) )/((2k+1)^2 )).((√2)/2)+Σ_(k≥1) (((−1)^k )/(4k^2 ))+iΣ_(k≥0) ((√2)/2).(1/((2k+1)^2 )) =Σ_(k≥0) (1/((2k+1)^2 )).((√2)/2)+Σ_(k≥1) (((−1)^k )/(4k^2 ))+(i/(√2))Σ_(k≥0) (1/((2k+1)^2 )) =((√2)/2)((π^2 /8))+(1/(16)).(π^2 /6)−(π^2 /(32))+(i/(√2)).(π^2 /8) =((π^2 (√2))/(16))−(π^2 /(48))+((iπ^2 )/(8(√2)))=(((3(√2)−1)π^2 )/(48))+((iπ^2 )/(8(√2)))

$$\mathrm{1}+{i}=\sqrt{\mathrm{2}}{e}^{{i}\frac{\pi}{\mathrm{4}}} \Rightarrow \\ $$$$=\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{\left(\sqrt{\mathrm{2}}\right)^{{k}^{\mathrm{2}} } {e}^{{i}\frac{{k}^{\mathrm{2}} \pi}{\mathrm{4}}} }{\left(\sqrt{\mathrm{2}}\right)^{{k}^{\mathrm{2}} } {k}^{\mathrm{2}} } \\ $$$$=\underset{{k}\geqslant\mathrm{1}} {\sum}\left({cos}\left(\frac{{k}^{\mathrm{2}} \pi}{\mathrm{4}}\right)+{isin}\left(\frac{{k}^{\mathrm{2}} \pi}{\mathrm{4}}\right)\right).\frac{\mathrm{1}}{{k}^{\mathrm{2}} } \\ $$$$=\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{{cos}\left(\frac{{k}^{\mathrm{2}} \pi}{\mathrm{4}}\right)}{{k}^{\mathrm{2}} }+{i}\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{{sin}\left(\frac{{k}^{\mathrm{2}} \pi}{\mathrm{4}}\right)}{{k}^{\mathrm{2}} } \\ $$$$=\underset{{k}=\mathrm{0}} {\overset{+\infty} {\sum}}\frac{{cos}\left(\left({k}^{\mathrm{2}} \pi+{k}\pi+\frac{\pi}{\mathrm{4}}\right)\right)}{\left(\mathrm{2}{k}+\mathrm{1}\right)^{\mathrm{2}} }+\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{{cos}\left({k}^{\mathrm{2}} \pi\right)}{\mathrm{4}{k}^{\mathrm{2}} }+{i}\underset{{k}=\mathrm{0}} {\sum}\frac{{sin}\left({k}^{\mathrm{2}} \pi+{k}\pi+\frac{\pi}{\mathrm{4}}\right)}{\left(\mathrm{2}{k}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}\left({k}+\mathrm{1}\right)} }{\left(\mathrm{2}{k}+\mathrm{1}\right)^{\mathrm{2}} }.\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}} }{\mathrm{4}{k}^{\mathrm{2}} }+{i}\underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}.\frac{\mathrm{1}}{\left(\mathrm{2}{k}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\mathrm{1}}{\left(\mathrm{2}{k}+\mathrm{1}\right)^{\mathrm{2}} }.\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}} }{\mathrm{4}{k}^{\mathrm{2}} }+\frac{{i}}{\sqrt{\mathrm{2}}}\underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\mathrm{1}}{\left(\mathrm{2}{k}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\left(\frac{\pi^{\mathrm{2}} }{\mathrm{8}}\right)+\frac{\mathrm{1}}{\mathrm{16}}.\frac{\pi^{\mathrm{2}} }{\mathrm{6}}−\frac{\pi^{\mathrm{2}} }{\mathrm{32}}+\frac{{i}}{\sqrt{\mathrm{2}}}.\frac{\pi^{\mathrm{2}} }{\mathrm{8}} \\ $$$$=\frac{\pi^{\mathrm{2}} \sqrt{\mathrm{2}}}{\mathrm{16}}−\frac{\pi^{\mathrm{2}} }{\mathrm{48}}+\frac{{i}\pi^{\mathrm{2}} }{\mathrm{8}\sqrt{\mathrm{2}}}=\frac{\left(\mathrm{3}\sqrt{\mathrm{2}}−\mathrm{1}\right)\pi^{\mathrm{2}} }{\mathrm{48}}+\frac{{i}\pi^{\mathrm{2}} }{\mathrm{8}\sqrt{\mathrm{2}}} \\ $$

Commented by M±th+et£s last updated on 20/Apr/20

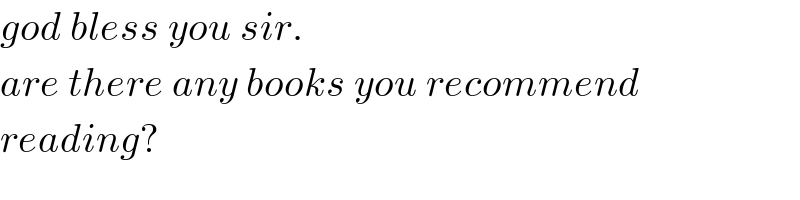
god bless you sir. are there any books you recommend reading?

$${god}\:{bless}\:{you}\:{sir}. \\ $$$${are}\:{there}\:{any}\:{books}\:{you}\:{recommend} \\ $$$${reading}? \\ $$

Commented by maths mind last updated on 20/Apr/20

Almost impposible Series and integral i will chek the right titel and comback

$${Almost}\:{impposible}\:{Series}\:{and}\:{integral}\: \\ $$$${i}\:{will}\:{chek}\:{the}\:{right}\:{titel}\:{and}\:{comback}\: \\ $$

Commented by M±th+et£s last updated on 20/Apr/20

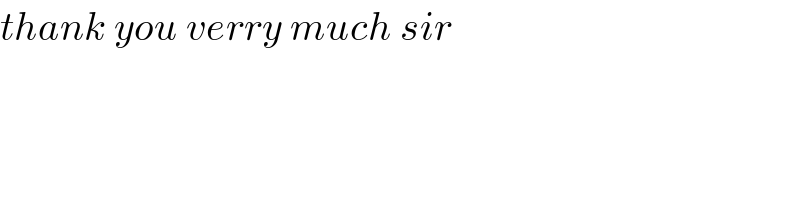
thank you verry much sir

$${thank}\:{you}\:{verry}\:{much}\:{sir}\: \\ $$

Commented by M±th+et£s last updated on 20/Apr/20

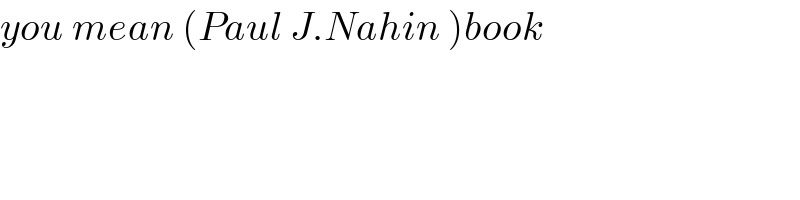
you mean (Paul J.Nahin )book

$${you}\:{mean}\:\left({Paul}\:{J}.{Nahin}\:\right){book} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com