Menu Close

lim-x-pi-2-ln-sin-2-x-pi-2-x-2-

Tinku Tara 0 Comments
Pin




Question Number 66316 by mathmax by abdo last updated on 12/Aug/19
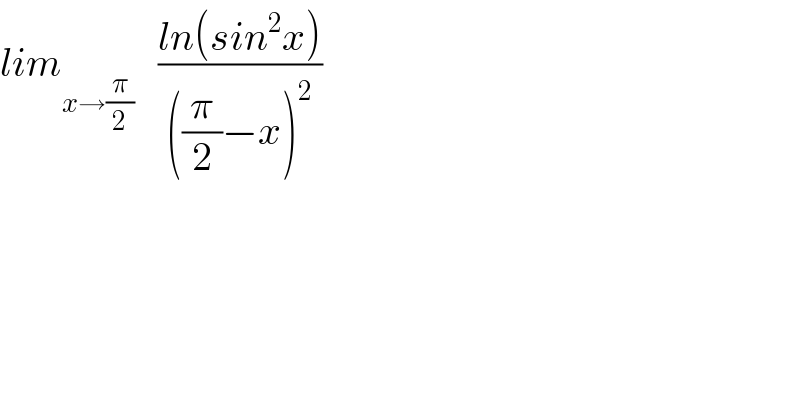
lim_(x→(π/2)) ((ln(sin^2 x))/(((π/2)−x)^2 ))
$${lim}_{{x}\rightarrow\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{ln}\left({sin}^{\mathrm{2}} {x}\right)}{\left(\frac{\pi}{\mathrm{2}}−{x}\right)^{\mathrm{2}} } \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 21/Aug/19
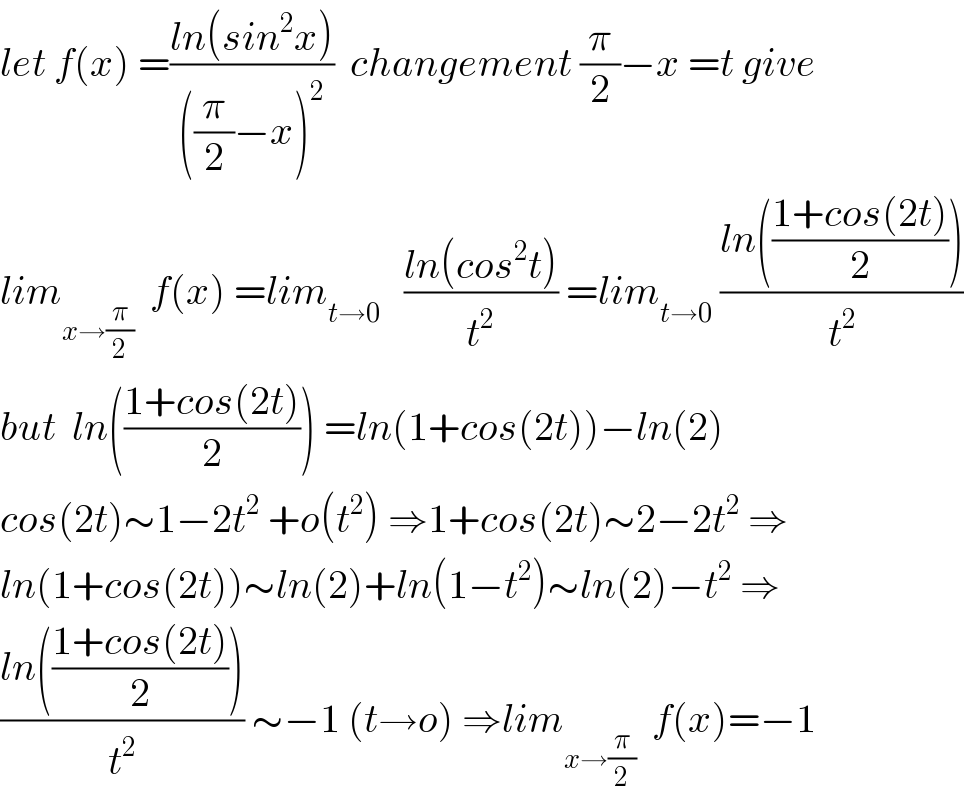
let f(x) =((ln(sin^2 x))/(((π/2)−x)^2 )) changement (π/2)−x =t give lim_(x→(π/2)) f(x) =lim_(t→0) ((ln(cos^2 t))/t^2 ) =lim_(t→0) ((ln(((1+cos(2t))/2)))/t^2 ) but ln(((1+cos(2t))/2)) =ln(1+cos(2t))−ln(2) cos(2t)∼1−2t^2 +o(t^2 ) ⇒1+cos(2t)∼2−2t^2 ⇒ ln(1+cos(2t))∼ln(2)+ln(1−t^2 )∼ln(2)−t^2 ⇒ ((ln(((1+cos(2t))/2)))/t^2 ) ∼−1 (t→o) ⇒lim_(x→(π/2)) f(x)=−1
$${let}\:{f}\left({x}\right)\:=\frac{{ln}\left({sin}^{\mathrm{2}} {x}\right)}{\left(\frac{\pi}{\mathrm{2}}−{x}\right)^{\mathrm{2}} }\:\:{changement}\:\frac{\pi}{\mathrm{2}}−{x}\:={t}\:{give} \\ $$$${lim}_{{x}\rightarrow\frac{\pi}{\mathrm{2}}} \:\:{f}\left({x}\right)\:={lim}_{{t}\rightarrow\mathrm{0}} \:\:\:\frac{{ln}\left({cos}^{\mathrm{2}} {t}\right)}{{t}^{\mathrm{2}} }\:={lim}_{{t}\rightarrow\mathrm{0}} \:\frac{{ln}\left(\frac{\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)}{\mathrm{2}}\right)}{{t}^{\mathrm{2}} } \\ $$$${but}\:\:{ln}\left(\frac{\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)}{\mathrm{2}}\right)\:={ln}\left(\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)\right)−{ln}\left(\mathrm{2}\right) \\ $$$${cos}\left(\mathrm{2}{t}\right)\sim\mathrm{1}−\mathrm{2}{t}^{\mathrm{2}} \:+{o}\left({t}^{\mathrm{2}} \right)\:\Rightarrow\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)\sim\mathrm{2}−\mathrm{2}{t}^{\mathrm{2}} \:\Rightarrow \\ $$$${ln}\left(\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)\right)\sim{ln}\left(\mathrm{2}\right)+{ln}\left(\mathrm{1}−{t}^{\mathrm{2}} \right)\sim{ln}\left(\mathrm{2}\right)−{t}^{\mathrm{2}} \:\Rightarrow \\ $$$$\frac{{ln}\left(\frac{\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)}{\mathrm{2}}\right)}{{t}^{\mathrm{2}} }\:\sim−\mathrm{1}\:\left({t}\rightarrow{o}\right)\:\Rightarrow{lim}_{{x}\rightarrow\frac{\pi}{\mathrm{2}}} \:\:{f}\left({x}\right)=−\mathrm{1} \\ $$
Answered by kaivan.ahmadi last updated on 12/Aug/19
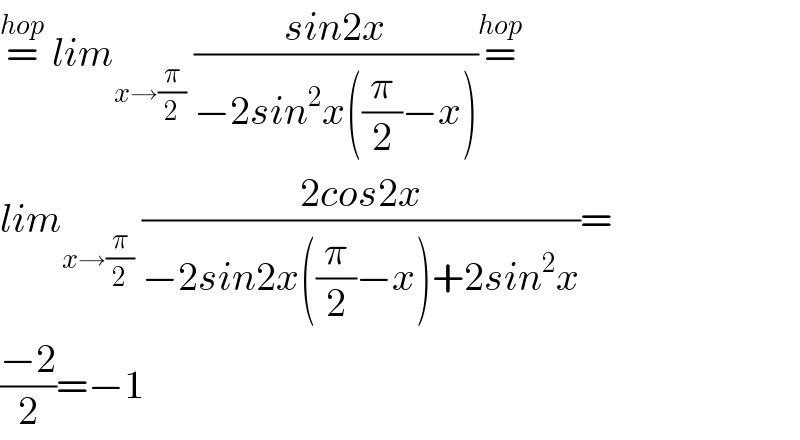
=^(hop) lim_(x→(π/2)) ((sin2x)/(−2sin^2 x((π/2)−x)))=^(hop) lim_(x→(π/2)) ((2cos2x)/(−2sin2x((π/2)−x)+2sin^2 x))= ((−2)/2)=−1
$$\overset{{hop}} {=}\:{lim}_{{x}\rightarrow\frac{\pi}{\mathrm{2}}} \:\frac{{sin}\mathrm{2}{x}}{−\mathrm{2}{sin}^{\mathrm{2}} {x}\left(\frac{\pi}{\mathrm{2}}−{x}\right)}\overset{{hop}} {=} \\ $$$${lim}_{{x}\rightarrow\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{2}{cos}\mathrm{2}{x}}{−\mathrm{2}{sin}\mathrm{2}{x}\left(\frac{\pi}{\mathrm{2}}−{x}\right)+\mathrm{2}{sin}^{\mathrm{2}} {x}}= \\ $$$$\frac{−\mathrm{2}}{\mathrm{2}}=−\mathrm{1} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *