Question Number 76721 by Rio Michael last updated on 29/Dec/19
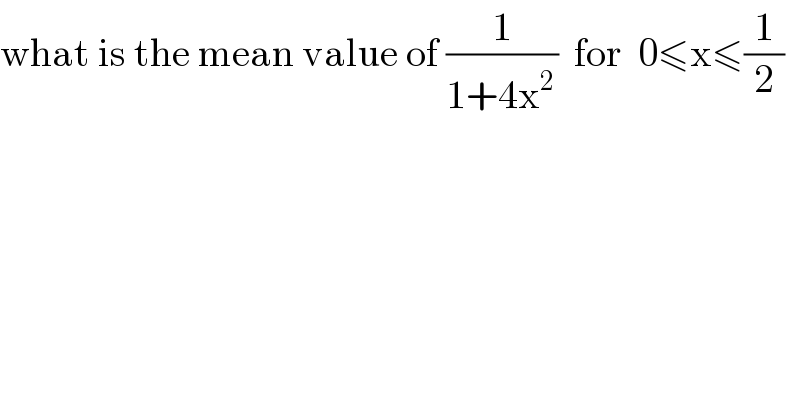
$$\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{mean}\:\mathrm{value}\:\mathrm{of}\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{4x}^{\mathrm{2}\:} }\:\:\mathrm{for}\:\:\mathrm{0}\leqslant\mathrm{x}\leqslant\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Answered by MJS last updated on 30/Dec/19
![mean (f(x)) with a≤x≤b (1/(b−a))∫_a ^b f(x)dx we′re calculating the area first and then the height of a rectangle of the width of our interval (1/((1/2)−0))∫_0 ^(1/2) (dx/(1+4x^2 ))=2∫_0 ^(1/2) (dx/(1+4x^2 ))= [t=2x → dx=(1/2)dt] =∫_0 ^1 (dt/(t^2 +1))=[arctan t]_0 ^1 =(π/4)](https://www.tinkutara.com/question/Q76731.png)
$$\mathrm{mean}\:\left({f}\left({x}\right)\right)\:\mathrm{with}\:{a}\leqslant{x}\leqslant{b} \\ $$$$\frac{\mathrm{1}}{{b}−{a}}\underset{{a}} {\overset{{b}} {\int}}{f}\left({x}\right){dx} \\ $$$$\mathrm{we}'\mathrm{re}\:\mathrm{calculating}\:\mathrm{the}\:\mathrm{area}\:\mathrm{first}\:\mathrm{and}\:\mathrm{then} \\ $$$$\mathrm{the}\:\mathrm{height}\:\mathrm{of}\:\mathrm{a}\:\mathrm{rectangle}\:\mathrm{of}\:\mathrm{the}\:\mathrm{width}\:\mathrm{of} \\ $$$$\mathrm{our}\:\mathrm{interval} \\ $$$$\frac{\mathrm{1}}{\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{0}}\underset{\mathrm{0}} {\overset{\frac{\mathrm{1}}{\mathrm{2}}} {\int}}\frac{{dx}}{\mathrm{1}+\mathrm{4}{x}^{\mathrm{2}} }=\mathrm{2}\underset{\mathrm{0}} {\overset{\frac{\mathrm{1}}{\mathrm{2}}} {\int}}\frac{{dx}}{\mathrm{1}+\mathrm{4}{x}^{\mathrm{2}} }= \\ $$$$\:\:\:\:\:\left[{t}=\mathrm{2}{x}\:\rightarrow\:{dx}=\frac{\mathrm{1}}{\mathrm{2}}{dt}\right] \\ $$$$=\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{{dt}}{{t}^{\mathrm{2}} +\mathrm{1}}=\left[\mathrm{arctan}\:{t}\right]_{\mathrm{0}} ^{\mathrm{1}} =\frac{\pi}{\mathrm{4}} \\ $$
Answered by john santu last updated on 30/Dec/19
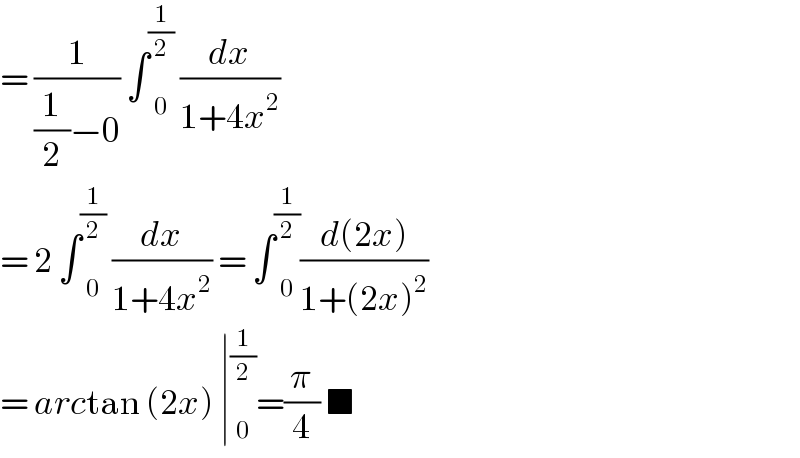
$$=\:\frac{\mathrm{1}}{\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{0}}\:\int\underset{\mathrm{0}} {\overset{\frac{\mathrm{1}}{\mathrm{2}}} {\:}}\:\frac{{dx}}{\mathrm{1}+\mathrm{4}{x}^{\mathrm{2}} } \\ $$$$=\:\mathrm{2}\:\int\underset{\mathrm{0}} {\overset{\frac{\mathrm{1}}{\mathrm{2}}} {\:}}\:\frac{{dx}}{\mathrm{1}+\mathrm{4}{x}^{\mathrm{2}} }\:=\:\int\underset{\mathrm{0}} {\overset{\frac{\mathrm{1}}{\mathrm{2}}} {\:}}\frac{{d}\left(\mathrm{2}{x}\right)}{\mathrm{1}+\left(\mathrm{2}{x}\right)^{\mathrm{2}} } \\ $$$$=\:{arc}\mathrm{tan}\:\left(\mathrm{2}{x}\right)\:\mid\underset{\mathrm{0}} {\overset{\frac{\mathrm{1}}{\mathrm{2}}} {\:}}=\frac{\pi}{\mathrm{4}}\:\blacksquare \\ $$
Commented by Rio Michael last updated on 30/Dec/19

$$\mathrm{thanks}\: \\ $$
